题目内容

输入一个n行m列的整数矩阵，再输入q个操作，每个操作包含五个整数x1, y1, x2, y2, c，其中(x1, y1)和(x2, y2)表示一个子矩阵的左上角坐标和右下角坐标。
每个操作都要将选中的子矩阵中的每个元素的值加上c。
请你将进行完所有操作后的矩阵输出。

输入格式

第一行包含整数n,m,q。
接下来n行，每行包含m个整数，表示整数矩阵。
接下来q行，每行包含5个整数x1, y1, x2, y2, c，表示一个操作。

输出格式

共 n 行，每行 m 个整数，表示所有操作进行完毕后的最终矩阵。

数据范围

1≤n,m≤1000 ,
1≤q≤100000,
1≤x1≤x2≤n,
1≤y1≤y2≤m,
−1000≤c≤1000,
−1000≤矩阵内元素的值≤1000

输入样例

3 4 3
1 2 2 1
3 2 2 1
1 1 1 1
1 1 2 2 1
1 3 2 3 2
3 1 3 4 1

输出样例

2 3 4 1
4 3 4 1
2 2 2 2

题解

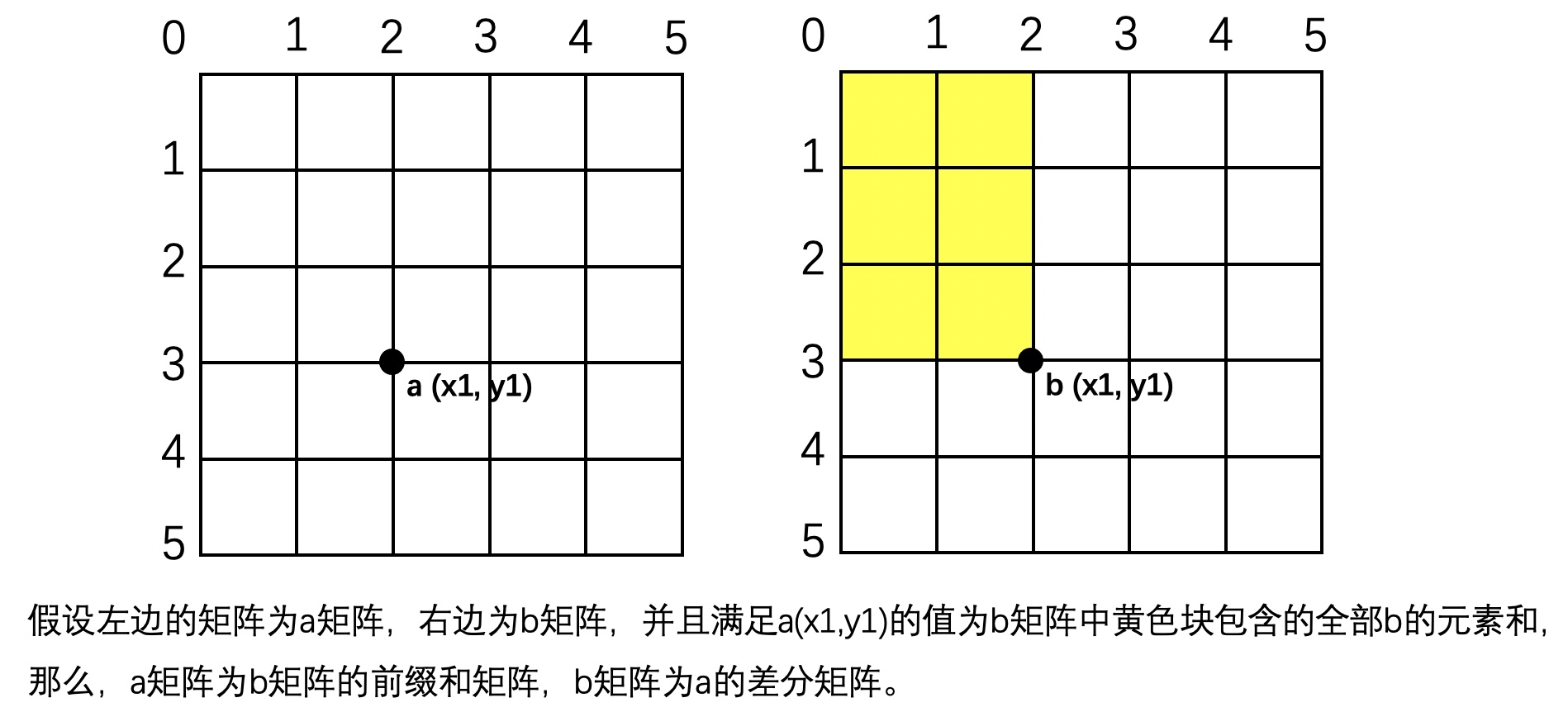
我们将题目中输入的矩阵看做a矩阵，那么只需要构造一个类似b矩阵的差分矩阵，那么此题的答案的时间复杂度即为O(1)。

代码

#include <iostream>

using namespace std;

const int N = 1010;

int n,m,q;
int a[N][N], b[N][N];

void insert(int x1, int y1, int x2, int y2, int c){
    b[x1][y1] += c;
    b[x2+1][y1] -= c;
    b[x1][y2+1] -= c;
    b[x2+1][y2+1] += c;
}

int main(){
    scanf("%d%d%d", &n,&m,&q);
    for(int i = 1; i <=n; i++)
        for( int j = 1; j<=m; j++)
            scanf("%d",&a[i][j]);
    for(int i = 1; i <=n; i++)
        for( int j = 1; j<=m; j++)
            insert(i,j,i,j,a[i][j]);
    while(q--){
        int x1,y1,x2,y2,c;
        cin >> x1 >> y1 >> x2 >> y2 >> c;
        insert(x1,y1,x2,y2,c);
    }
    
    for(int i = 1; i<=n; i++)
        for (int j = 1; j<=m; j++)
            b[i][j] += b[i-1][j] + b[i][j-1] - b[i-1][j-1];
    for(int i = 1;i<=n;i++){
        for(int j=1 ;j<=m; j++) printf("%d ", b[i][j]);
        puts("");
    }
    return 0;
}

原题链接